8.F.A.3. Interpret the equation $y = mx + b$ as defining a linear function, whose graph is a straight line; give examples of functions that are not linear. For example, the function $A = s^2$ giving the area of a square as a function of its side length is not linear because its graph contains the points $(1,1)$, $(2,4)$ and $(3,9)$, which are not on a straight line.
I could write a whole blog post about that comma in the first sentence, but for now I want to focus on the question of what exactly are the student expectations entailed by this standard. Certainly students should be able to recognize that $y = mx + b$ defines a linear function; and they should be able to show a function is not linear by finding points on the graph with different slopes between them. (By definition, a linear function is one with a constant rate of change, that is, a function where the slope between any two points on its graph is always the same.) However, the following PARCC released item suggests the possible expectation that students be able to tell if a function is linear or not purely from looking at its defining equation.
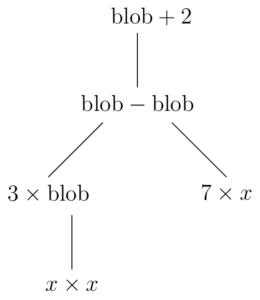
PARCC Released Item Grade 8
My guess is that the writer of this item was thinking that students would detect non-linear functions by noticing the functions with squared and cubed terms (they could also use the point method but that doesn’t seem likely). That’s not a good path to lead them down. Sure, $y = 5-x^2$ is not linear. What about $y = 5 – x^2 + (x+1)^2$? By the look of it it is even more not linear! But of course it is in fact linear, because the expression on the right is equivalent to $2x + 6$. The grade 8 standards don’t expect students to be making such simplifications as expanding squares of binomials.
This is another example of the confusion between expressions and functions. The expression $(x+1)^2 – x^2$ has non-linear terms in it, but the function it defines is linear because it is equivalent to $2x + 1$. Equivalent expressions define the same function.
There’s another confusion revealed in this item, the confusion between equations and functions. Look at option C. Is it intended to be a distractor? Will a student who chooses it be marked wrong? Such a student would have a case for protest on the grounds that $-3x + 2y = 4$ is not a linear function because it is not a function, it is an equation. Certainly if you choose to think of $x$ as the input and solve for $y$ to get the output you can think of it as a function, which would indeed be linear. You could also go the other way around and choose $y$ as the input and get a different linear function. It is conventional when $x$s and $y$s are floating around to think of $x$ as the input and $y$ as the output, but you can flout convention without being mathematically incorrect.
The moral of this story is, I suppose, that it is easier to tell when a function is not linear than to tell when it is linear. Testing for non-linearity involves just picking a few points on the graph; testing for linearity involves picking every possible pair of points on the graph and verifying that the slope between them is always the same. It’s instructive to do this with $y = mx +b$: if $(x_1,y_1)$ and $(x_2,y_2)$ are two different ordered pairs satisfying this equation, then $$\frac{y_2-y_1}{x_2-x_1} = \frac{{mx_2 + b} – (mx_1 +b)}{x_2-x_1} = \frac{m(x_2-x_1)}{x_2-x_1} = m.$$
OK, next week I’ll write a blog post about the comma.
 .
.