In our last post, we asked people if they could come up with a division story problem for $1\frac{3}{4}÷\frac{1}{2}$. Interestingly, almost all of the responses used the “how many groups?” interpretation of division. When interpreting multiplication in terms of groups, the two factors play different roles, and so there is another interpretation of division worth exploring.

If we say that $a\times b$ means $a$ groups of $b$, then

• a division situation where $b$ and $a\times b$ are known but $a$ is unknown is called a “how many groups?” division problem
• a division situation where $a$ and $a\times b$ are known but $b$ is unknown is called a “how many (or how much) in each (or in one) group?” division problem.

[Pause here and see if you can come up with a “how much in one group?” story problem for $1\frac{3}{4}÷\frac{1}{2}$.]

How do these two interpretations of division come into play as students learn about fraction division? In grade 5, students solve problems like $6÷\frac{1}{2}$ and $\frac{1}{2}÷6$. What’s nice about problems involving a whole number divided by a unit fraction or a unit fraction divided by a whole number is that we can think of them using the same structure that we thought about division of whole numbers.

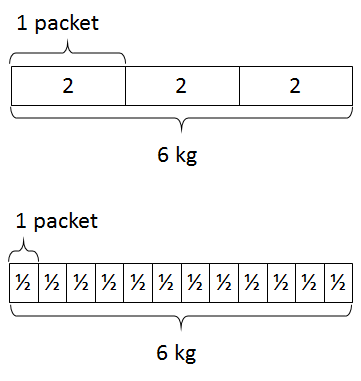
1. Kiki has 6 kg of chocolate chips. How many 2 kg packets of chocolate chips can she make?
2. Kiki has 6 kg of chocolate chips. How many $\frac{1}{2}$ kg packets of chocolate chips can she make?

Notice that these are both a “how many groups?” division problem, and because there is always a whole number of unit fractions in 1, the solution will be a whole number of groups (so students do not have to worry about fractions of a group). If we write equations to represent these problems, that can also help us see the structure:

$$?\times 2=6$$

$$?\times \frac{1}{2}=6$$

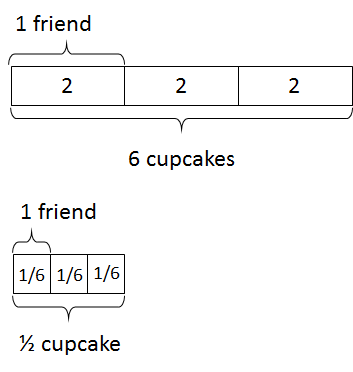
3. Nero had 6 cupcakes and 3 friends he wanted to share them with equally. How many cupcakes does each friend get?
4. Nero had $\frac{1}{2}$ of a cupcake and 3 friends he wanted to share them with equally. How many cupcakes does each friend get?

Notice that these are both a “how many in each (or how much in one) group?” division problem, and students don’t have to worry about fractional groups because the whole number in the problem is the number of groups.

Again, with equations:

$$3\times ?=6$$

$$3\times ?=\frac{1}{2}$$

So in grade 5, students can build on their understanding of whole number division without having to grapple with fractional groups, so long as they understand both of these interpretations of division.

In grade 5, students also learn about fraction multiplication, so they do encounter fractions of a group, but they are not required to put these two understandings together until grade 6 when they extend their understanding of division to all fractions. This provides some scaffolding for students on their way to understanding division of fractions in general.

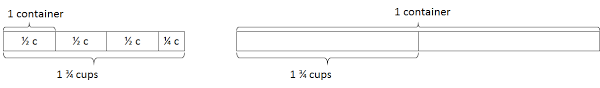
Let’s look at these two interpretations of division for $1\frac{3}{4}÷\frac{1}{2}$.

• “How many groups?” : I need $1\frac{3}{4}$ cups flour, but I only have a $\frac{1}{2}$ cup measure. How many times do I have to fill the $\frac{1}{2}$ measure to get $1\frac{3}{4}$ cups flour? (A version of this was suggested by two different people on our last post.)
• “How much in one group?” : I have a container with $1\frac{3}{4}$ cups flour. The container is $\frac{1}{2}$ full. How much flour does the container have when it is full?

Here are two possible diagrams to represent these two interpretations of division:

Next time: how the different interpretations of division and diagrams can be used to understand the “invert and multiply rule” and other approaches to understanding this procedure.