There’s been a lot of talk recently about the Common Core and drawings. I won’t get into the politics of that here—that’s for my other blog—but, politics aside, there is an important question of interpretation, and possible misinterpretation, of the standards here. Let’s look at the wording of standards that talk about drawings.
First I want to draw attention to a footnote on K.OA.A.1, the first standard in which drawings are mentioned, in Kindergarten. The footnote says
Drawings need not show details, but should show the mathematics in the problem. (This applies wherever drawings are mentioned in the Standards.)
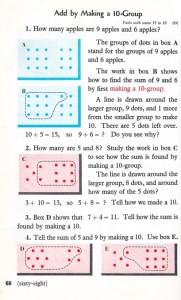
Not all drawings “show the mathematics in the problem.” Here’s an example of one that does, courtesy of Barry Garelick, who has written a very interesting article about how the Common Core can support a traditional approach to teaching (you have to scroll to the second section to get to the math). It is from a 1955 textbook by Brownell et al.
The arrangement of dots and the enclosing curve have a clear purpose here in helping students learn to add by making a 10; the drawing has a mathematical purpose, it is not just a random collection of dots.
In Grade 2 we have:
2.OA.A.1. Use addition and subtraction within 100 to solve one- and two-step word problems involving situations of adding to, taking from, putting together, taking apart, and comparing, with unknowns in all positions, e.g., by using drawings and equations with a symbol for the unknown number to represent the problem. [Bold face added.]
Some form of the boldfaced wording in this standard occurs repeatedly in the K–5 standards. The intended interpretation of this wording (e.g the “e.g.”) is to indicate that drawings are one possible way of learning a strategy and of understanding why it works. It is not intended that every single problem be accompanied by a drawing and an equation. Moreover, there is a progression in the standards, culminating in requirements that students be fluent with the standard algorithms. So there should be a progression in the use of drawings, with students eventually being able to do in their heads what they first had to do on paper, and with them eventually acquiring a robust fluency that does not require them to spell out their understanding explicitly every time.
This progression is clearly indicated by the following progression of standards about addition and subtraction from Grade 2 to Grade 4:
2.NBT.B.7 Add and subtract within 1000, using concrete models or drawings and strategies based on place value, properties of operations, and/or the relationship between addition and subtraction; relate the strategy to a written method. Understand that in adding or subtracting three-digit numbers, one adds or subtracts hundreds and hundreds, tens and tens, ones and ones; and sometimes it is necessary to compose or decompose tens or hundreds.
3.NBT.A.2. Fluently add and subtract within 1000 using strategies and algorithms based on place value, properties of operations, and/or the relationship between addition and subtraction.
4.NBT.B.4. Fluently add and subtract multi-digit whole numbers using the standard algorithm.
If you read the standards carefully for the progressions in them you can see the clear intention about drawings. They have their place on the pathway to mathematical proficiency, but are not an end in themselves … and should not become an obsessive requirement.
By the way, the standard algorithms for addition and subtraction are “strategies based on place value, properties of operations, and/or the relationship between addition and subtraction.” The standards do not forbid them being introduced in Grade 2—nor do they require that.
I’ve gone on enough for now. Maybe I’ll do another post on drawings and fractions when I get a chance.